All ISOPHOT detectors are photoconductors. Therefore, the crucial calibration measure is the photocurrent generated by the incident infrared photons. A detector is characterised by the detector responsivity $R_{det}$ which is the ratio of the photocurrent

and the in-band power $P_{src}$ :

In the first stage of data collection the output voltage of an integrating amplifier is measured. From these data the signal or voltage increase per unit time is obtained, see Section 2.4.2. The in-band power can be derived from signal and responsivity, if the capacitance of the integration capacitor, $C_{int}$ , is known:

where $s_{src}$ is the source signal in V/s. The value for $C_{int}$ depends on the detector used and is assumed to be constant throughout the mission. $R_{det}$ , however, is not a constant and can depend on external parameters such as the strength of the ionising radiation and flux history.

In principle, fine calibration source (FCS) measurements scheduled in all PHT-P and PHT-C AOTs should ensure that $P_{src}$ can directly be obtained from the comparison between the sky and the FCS signal without knowledge of the exact value of $R_{det}$ .

FCS measurements are obtained in all ISOPHOT AOTs, except PHT40. However, for some observations the FCS measurements can be of insufficient quality, or, completely absent due to technical problems (e.g. telemetry failure). In such cases a default responsivity should be adopted.

The default detector responsivities were derived during the in-orbit calibration. The values of the default responsivities are provided in Cal-G files, see Section 14.13. The relationships between FCS heating power and the corresponding in-band power measured by the different detectors are also given in Cal-G files.

The above description of the detector responsivity is still idealised and is only valid for responsivity values averaged over time scales longer than the measurement time. On shorter time scales the responsivity can still vary. The stability of the responsivity depends on the radiation history including the history of ionisation radiation. Detailed descriptions of the most important phenomena are presented in the next sections.

In case of a PHT-S observation with AOT PHT40 no FCS measurement is performed (see Section 2.7). The PHT-S signals are directly converted to flux densities. The Cal-G files containing the conversion factors for each PHT-S pixel are described in Section 14.19. The conversion factors for each pixel have been derived from the signals of several calibration stars.

4.2.2 Signal non-linearities

A detector is linear, if the photocurrent and hence the resulting output signal is proportional to the incident flux, i.e. the corresponding in-band power. Deviations from linearity introduce a bias when deriving the responsivity from the FCS measurement or when using a single (default) value for $R_{det}$ . To avoid this bias the FCS signal is tuned close to the source signal by the AOT logic. This strategy may fail in the following cases:

In these cases a wide range of signals is produced during the sky measurements, whereas the calibration is performed by one or more FCS measurements yielding a single signal level at the maximum or mean of the range.

In-orbit calibration observations showed that the detector responsivity depends on the infrared flux falling on the detector. The responsivity $R_{det}$ is a function of the photocurrent or signal $s = s_{src}+s_{bck}$ which consists of a source ( $s_{src}$ ) and background ( $s_{bck}$ ) component. Following Equation 4.2, the responsivity of the detector when it is pointed at the position of the source can be written:

$\begin{displaymath} R(s_{src}+s_{bck}) = \frac {(s_{src}+s_{bck})C_{int}} {P... ...ac {s_{bck}C_{int}}{R(s_{bck})}} ~~~~~~~~~~~~~~~~[{\rm W/A}] \end{displaymath}$

(4.3)

In practice, $R(s_{src}+s_{bck})$ and $R(s_{bck})$ are not necessarily equal and the ratio between the signals measured on- and off-source is different from the respective in-band powers. The discrepancy is larger if very different source and background signals are compared, like for the cases listed above.

For the ISOPHOT photometric calibration a linear system is obtained by linearising the detector signals,

, according to:

The condition for

is that

inserted in $R(s') = s'C_{int}/P$ yields a constant responsivity, see also Section 5.2.2 for more details on the correction.

4.2.3 Transient behaviour after flux change

After an illumination change, the output signal of a PHT detector shows a systematic drift in time. Such a drift effect is also referred to as a detector transient. A common feature to all transient curves is the asymptotic approximation to a stable level. This typical slow response is due to the presence of low ohmic contact material necessary to connect the detector substrate with the metallic wires.

Typical drift curves are presented in Figure 4.1. In case of a flux drop, a signal decay and in case of increasing flux steps a signal rise (Figure 4.1) is observed. The doped silicon detectors (SS, SL, P1, and P2) exhibit a hook response during the first 40 seconds after large positive flux steps (Figure 4.1b). The signal shows a behaviour similar to a strongly damped oscillation around the asymptotic level. For even higher flux steps the signal behaviour can be restricted to an overshoot followed by a slow decay.

**Figure 4.1:** Examples of P1 detector transients. Left panel (A): 4 transient curves after a flux step overplotted in the same graph for comparison, the highest curve corresponds to the largest positive flux step. In all cases the signal prior to each step was the same and had a strength of about 35 V/s. For these flux steps a signal overshoot is detected followed by a strongly damped oscillation. Right panel (B): multi-filter observation of the star HR6705, filter sequence P_16 (64 s integration), P_3.6 (64 s) followed by the P_3.6 (32 s) FCS measurement. Both the P_16 as well as the P_3.6 FCS measurement show a downward drift, with a longer stabilisation time for the fainter P_16 signal.
$\resizebox {14cm}{!}{\includegraphics{driftfig1.eps}}$

The characteristic stabilisation time scales depend on (1) the detector material, (2) the flux change, (3) the illumination level, and (4) the temperature of the detector. They vary from a few seconds up to several minutes.

Typical time scales for stabilisation after a given flux step for the PHT-SS/SL detectors are presented in Table 4.1. These time scales can be taken as approximate values for detector P2 and lower limits for P1. The stabilisation time is defined as the time after a flux step to reach 90% of the signal at $t=\infty$ ( $S_{\infty}$ ).

Note that a signal of 0.5 mV/s is very close to the dark signal and - in practice - cannot be determined accurately. The relative stabilisation time is faster for positive flux steps - see Table 4.1 values below the diagonal versus those above the diagonal. Flux steps at low flux levels will take more time to stabilise. However, in such cases the S/N and the detector stability (see next section) might be more important.

The doped germanium detectors P3 and C100 tend to stabilise faster than the doped silicon detectors. C200 shows the shortest transient time scales. Typical time scales are 100 s for P3 and C100 and 40 s for C200, respectively.

**Table 4.1:** *Stabilisation times for a given flux step in seconds. The first row lists the initial signal, the first column the final signal in V/s.*
	5 10 $^{-4}$	0.001	0.01	0.1	1	10
5 10 $^{-4}$	...	6800	11500	11880	11900	11900
0.001	4070	...	5570	5900	5960	5960
0.01	1500	1220	...	560	592	595
0.1	240	210	122	...	55	59
1	32	30	21	12	...	5
10	4	4	3	2	1	...

For chopped measurements the same drift behaviour description applies. Due to the chopper modulation, the signal evolution on each chopper plateau can be regarded as a drift curve after a flux step. Consequently, the difference signal between the on- and off-target chopper plateaux is not only a function of flux step, but also a function of chopper frequency. Without applying drift corrections use of the difference signal seriously underestimates the actual flux of the target. It has been observed that the signal differences between neighbouring chopper plateaux remain stable, even in case of a measurement containing a general drift (see Section 4.2.4), provided that the flux difference between the chopper plateaux is less than 50% of the total signal. An example of a chopped measurement with P2 is presented in Figure 4.2.

PHT32 uses the chopper in the sawtooth mode to obtain oversampled maps. Detector transients of bright sources can introduce ghost images in neighbouring areas. This happens when a bright source was observed at the end of a sawtooth sweep. When the chopper mirror was directed back to the starting point of the sweep, the transient caused an increased signal which was recorded at the beginning of the next chopper sweep. The signal decays with the relevant time scale thereby mimicing a point source detection. Consequently, the ghost is always displaced from the bright source by

(=amplitude of the chopper sweep) in Y-direction.

The study of transients led to the development of a PHT AOT logic which should minimise disturbing effects by detector transients:

Not every measurement lasted long enough to provide a stabilised signal. Laboratory and in-flight measurements showed that the transient curves were reproducible under the same flux conditions. Methods to correct for these transient drifts based on physical models as well as on empirical results have been developed.

A description of detector transients tested against the doped silicon detectors of PHT-S can be found in Schubert et al. 1995, [49]. C200 detector transients have been studied by Wilke 1995, [56]. A study of in-orbit transients and descriptions of correction methods and practical recipes are presented in Acosta-Pulido, Gabriel & Castañeda 2000, [3].

**Figure 4.2:** Examples of P2 detector transients induced by the chopper modulation on a very high source-background contrast. Left panel (A): slow (32 s per chopper plateau) chopped measurement on a high source-background contrast. Right panel (B) same source-background contrast as under (A) but with a a higher chopper frequency (8 s per plateau). Note the strong overshoot in the first higher plateau; a straight signal average per chopper plateau would underestimate the background subtracted source signal in both cases.
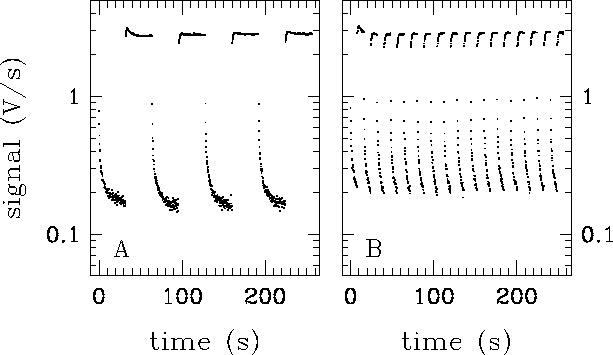
$\resizebox {14cm}{!}{\includegraphics{chopfig1.eps}}$

In OLP no sophisticated treatment of transients is applied. Instead, an algorithm is used which determines per chopper plateau whether a significant signal drift is present. In case such a drift is found, the last stable part of a chopper plateau is used (see also Section 7.3.5).

An alternative correction method is to perform the calibration measurement in the same way as the astronomical measurement of the unknown source and on a celestial standard of similar brightness. Then, the transients which show the same time scale and amplitude for both measurements cancel out in the calibration process. This method is succesfully applied for both chopped and staring PHT-S measurements.

4.2.4 Drift behaviour of responsivity

Besides the transient behaviour, the responsivity of a detector can vary or drift on time scales ranging from less than one hour up to the entire science window of 16 hours (see `ISO Handbook, Vol. I: ISO - Mission & Satellite Overview', [20]). Responsivity drifts are mainly observed for the doped germanium detectors (P3, C100 and C200) and are a result of both the illumination history of the detector as well as the rate of ionising radiation falling on the detector, see also Section 4.4.

Ionising radiation causes the detector responsivity to increase with time. This increase can be as high as 80%, 100% and 30% for P3, C100, and C200, respectively. The responsivity drift for these detectors is steeper at the end of the science window where the amount of ionising radiation rapidly increased. Long integrations with the same detector can be affected by this effect. Examples are multi-filter photometry with PHT22 and multi-aperture photometry with P3 in PHT04. For large (raster) maps available in AOTs PHT03, PHT22, PHT32, and in the sparse map modes PHT17/18/19 and PHT37/38/39, the responsivity drift can be assessed from the two FCS measurements collected at the beginning and end of each map. For polarisation observations the polarisers are cycled with a cycle time of 128 s per polariser to assess the long term stability of the detector during the observation.

On the other hand, very high flux levels in excess of 5 V/s for P3 and C100, and 10 V/s for C200 can cause a curing of the detector during the measurement (see also Section 4.2.5). The resulting effect is a decrease of the detector responsivity during the measurement. This decrease can amount to several percent up to a factor 1.5 of the initial responsivity. Such drifts are very difficult to correct for.

The observed responsivity drifts for the doped silicon detectors P1, P2 and PHT-SS/SL are less than 20% over the entire science window.

4.2.5 Curing procedures

Due to the high ionising radiation doses during perigee passage of ISO the responsivities and noise levels of the ISOPHOT detectors were strongly increased before the beginning of the new science window. Therefore, appropriate curing procedures were designed for the different detectors to restore the nominal responsivities. The procedures were applied after the switch-on of the instrument, before the beginning of the science window.

For the doped germanium detectors (P3, C100 and C200) a combination of bias boost (absolute increase of the bias voltage) and two to three infrared flashes using one of the FCSs were applied. For the doped silicon detectors (SS, SL, P1, and P2) curing was achieved by exposing the detector to a higher temperature at a reduced bias voltage for a defined period of time. In addition, P1 underwent an infrared flash curing.

The doped germanium detectors were much more susceptible to drifts caused by accumulating effects of the high energy radiation impacts (Section 4.4). In order to keep their responsivities within the nominal range a second curing procedure was applied around apogee passage in the handover window, when the satellite control was switched from VILSPA (Madrid) to Goldstone (California) (see `ISO Handbook, Vol. I: ISO - Mission & Satellite Overview', [20]). More about the effects of ionising radiation inside the science window is described in Section 4.4.

Trend analysis of responsivity measurements performed immediately after the curing procedures indicates that the nominal responsivities are re-established with $\pm$ 2% accuracy for all detectors, if the space environment conditions are stable.

The doped silicon detectors are not very sensitive to geomagnetic storms. The responsivity variations for PHT-SS, SL, P1, and P2 are presented in Figure 4.3. It shows that the responsivity could be restored to better than 2% of the mean value. A long term variation can be noticed which is possibly correlated with the position of the ISO orbit with respect to the asymmetric magnetic field of the Earth.

The doped germanium detectors are more sensitive to the space environment with variations in responsivity between 20-50% during geomagnetic storms. A striking illustration is given in Figure 4.3 where the P3, C100 and C200 responsivity variations over most of the ISO mission are shown. The detector responsivity peaks can last for several revolutions. They are correlated in time with the geomagnetic storm activity induced by solar flares.

An overview of the correlations between ISOPHOT detector responsivities and space weather parameters is presented in Castañeda and Klaas 2000, [5].

**Figure 4.3:** The results of the responsivity checks during instrument activation (AOT PHT83). From top to bottom, left to right, are the detectors: P1, P2, P3, C100 (average of 9 pixels), and C200 (average of 4 pixels), PHT-S pixel 120 (SL) and pixel 60 (SS, dashed). All the data are represented as relative change from an adopted mean; for the P1 and P2 detectors the mean is the average signal during the mission; for P3, C100, and C200, and PHT-S the reference is the mean signal for revolutions 192-212, a period of stable space weather (taken from [5]).
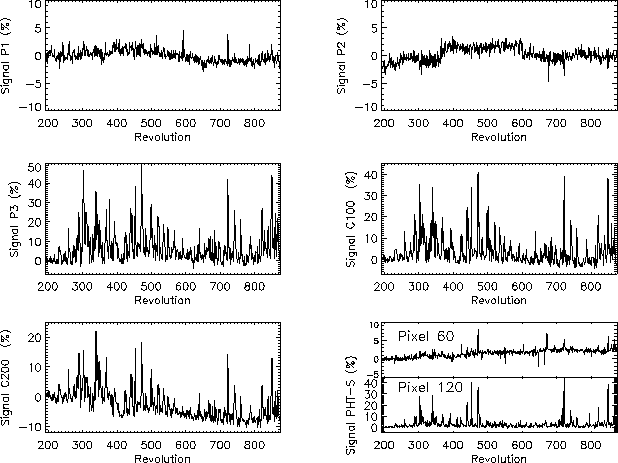
$\resizebox {14cm}{!}{\includegraphics{dettrends.eps}}$

4.2.6 Dark signal and noise

Dark current in the detector assembly adds a spurious dark signal to the source signal. Dark signals for the different detectors have been measured by means of dedicated observations in the dark instrument configuration (c.f. Table 3.1). These observations have been collected frequently throughout the mission and distributed over the science window. The in-orbit dark signals were found to be several factors higher than expected from pre-flight data. For all detectors except P3, the dark signals increased by a factor 2-3. For P3 the dark signal is up by a factor $\leq$ 50. The increase is attributed to the effects of ionising radiation on the detectors.

The typical equivalent fluxes to dark signals for a number of detector/filter combinations are listed in Table 4.2. A flat spectrum ( ${\nu}F_{\nu}=$ Constant) is assumed in the derivation of the dark flux values. In case one would like to know the typical dark flux for a different filter of the same detector one should multiply the values in the table with the factor C1(table_filter)/C1(new_filter) where C1 is the transmission parameter described in Section 5.2.5. The values in Table 4.2 were derived by assuming default responsivities for the detectors (given in Section 14.13). Note that pixel 6 of the C100 array shows a factor 3-7 higher dark signal than the other pixels in the C100 array.

**Table 4.2:** *Dark signal equivalent fluxes.*
Detector ID	Filter ID	Dark Flux
		[mJy]
P1	P_11p5	41
P2	P_25	410
P3	P_60	48
C100(1)	C_100	55
C100(2)	C_100	116
C100(3)	C_100	81
C100(4)	C_100	50
C100(5)	C_100	100
C100(6)	C_100	395
C100(7)	C_100	48
C100(8)	C_100	56
C100(9)	C_100	95
C200(1)	C_160	34
C200(2)	C_160	36
C200(3)	C_160	27
C200(4)	C_160	35

The pixels of the PHT-S arrays have dark signals that show systematic variations with wavelength (see Figure4.4). Improper dark signal subtraction could cause the presence of spurious features in the spectrum. The dark signals for a number of PHT-SS detectors can be negative, this is not due to a negative dark current but due to a CRE effect, see also Figure4.4. The equivalent flux to the dark signal in PHT-SL is typically 50 mJy for pixel 65 up to 250 mJy for pixel 128. For more details about the PHT-S characteristics, see Klaas et al. 1997, [23].

**Figure 4.4:** *Dark signals for both the PHT-SS and SL channels.*
$\resizebox {10cm}{!}{\includegraphics{phtsdarks.eps}}$

Trend analysis of dark calibration observations shows for all detectors a systematic variation as a function of orbital position. The dark signal for P2, C100 and C200 gradually rises by a few percent per hour, accumulating to nearly a factor of two towards the end of the revolution. Around 18 hours after perigee, in the last few hours of the science window, the dark signal steeply increases for all detectors except for P2. This effect was caused by the rapidly increasing amount of ionising radiation at the end of the science window. The germanium doped detectors (P3, C100 and C200) exhibit the largest increase of up to a factor 2-3. The dark signals for PHT-SL long wavelength pixels show a relatively high level at the beginning of the science window which is decreasing within the first few hours. This results from a memory of the bright illumination of SL during the responsivity check after curing in order to verify the proper performance of both S-arrays. Dark signal corrections depending on orbital position are available since OLP Version 7.

The dark signals can be higher for all detectors during a geomagnetic storm. If there are suspicions about the impact of space weather on the quality of an observation, it is advised to check the planetary `K-index'. This parameter is strongly correlated with the space weather conditions. If 48 hours before a given measurement or on the same day the K-index is larger than or equal to 4, it is possible that the measurement has been affected by the space weather. Tables of the K-index during the ISO mission are made available in the ISO Data Archive, see also Castañeda & Klaas 2000, [5].

For differential observations the dark signal cancels out, if the observations were taken close in time (

hour). Possible uncertainties in the target signal caused by the dark signal are in such cases automatically removed. For absolute photometry measurements, in which the total sky flux must be determined, the dark signal contribution can be important. These observing modes (PHT05 and PHT25) offered the possibility to include a dedicated dark signal measurement.

All standard observations with PHT-S (AOT PHT40) are preceded by a 32 s measurement in dark instrument configuration. This `pseudo dark' measurement cannot be used to subtract PHT-S dark signals, but offers the possibility to assess remaining detector transients from an earlier PHT-S observation, see Section 5.6.7.

Average dark signal values per orbital phase, obtained from many calibration observations are stored in a Cal-G file (see Section 14.7). These values are used to remove the dark signal from each measurement during SPD level processing.

4.2.7 Detector flat-fields

The pixels of the C100 and C200 arrays can be regarded as a number of individual detectors with their own responsivities. The detector flat-field gives the relative variation of the responsivites among the pixels with respect to the average responsivity over the array. The flat-fields measured in-orbit were found to be wavelength dependent. Therefore, for each C-detector/filter combination a separate flat-field is necessary.

For those observations where the associated FCS measurement is done with the same filter, the flat-field correction is included in the responsivity values derived from the FCS measurements after correction for illumination variations (see Section 4.5.4). However, observations with different filters compared to the FCS measurement need additional correction for the wavelength dependence of the flat-field. See also Section 7.10.4.

4.2.8 Detector saturation

This effect must not be confused with saturation of the readout electronics due to a wrong flux-estimate. The ISOPHOT detectors were specified for a certain flux range and laboratory measurements with replicas of the flight detectors demonstrated that up to $5 \times 10^{-10}$ W could be imposed onto the detectors. Nevertheless, a few celestial sources like IRC+10216, 07 Car, VY CMa and Jupiter could have imposed higher powers onto the detectors in some bandpasses or pixel ranges of P1 and PHT-S. As there was the potential danger that flooding these detectors with such a high photon rate could have led to a detector breakthrough with the detector becoming low ohmic, these targets were blocked for scheduling observations with P1 and PHT-S. Low ohmic detectors with a high bias voltage would have produced high currents onto the entrance of the sensitive readout electronics-multiplexer chain and would have irreversibly damaged them.

4.2 Detector Properties