


Next: 4.6 Point Spread Function
Up: 4. Calibration and Performance
Previous: 4.4 Transients
4.5 Flat-Fields
The flat-field can be defined as the relative response
of different pixels of the detector to a source with uniform
brightness distribution within the field of view.
For ISOCAM it is useful to distinguish between the detector
flat-field and the optical flat-field.
The detector flat-field can be defined
as the relative response of different pixels to a uniform
illumination of the detector, while the optical flat-field can be defined
as the relative flux falling onto different pixels when a source of
uniform brightness distribution occupies the field of view.
The observed flat-field results from the product of the detector
flat-field and the optical
flat-field. The need to separate the two arises because the position jitter
of the ISOCAM wheels (described in Section 4.11)
means that the
optical flat-field can move slightly over the detector.
While the detector flat-field is strictly related to the
relative sensitivity of the detector pixels to incoming flux, the
optical flat-field measures the vignetting due to the optical components of
the camera, in particular the aperture stops near the filters. The
vignetting profile is a function of the angular distance from the
optical axis, and with different lens magnifications the detector
sees different parts of the vignetting profile. Even with
the same lens, different optical flat-fields may arise, as mentioned
above, because of the wheel jitter
(see Section 4.11).
In practice, the detector flat-field is not directly observable. So, by
convention, the flat-field at 1.5
 is defined to be the detector flat-field for any given filter, because it
suffers less than other pfov flat-fields from vignetting effects. The
optical flat-fields at any pfov are obtained by dividing the observed
flat-fields by the
appropriate filter detector flat-field.
As a consequence, the optical flat-field
at 1.5
is defined to be the detector flat-field for any given filter, because it
suffers less than other pfov flat-fields from vignetting effects. The
optical flat-fields at any pfov are obtained by dividing the observed
flat-fields by the
appropriate filter detector flat-field.
As a consequence, the optical flat-field
at 1.5
 is a matrix with 1's everywhere.
Flat-fielding is a crucial step in the data processing, in particular
in the infrared, where the brightness of the background is very often
comparable to (or higher than) the brightness of the target
source. Therefore, the generation of flat-fields
is a very important step in
the overall calibration strategy of ISOCAM.
It must be noted,
however, that many observations in raster and beam-switch mode allow
the observer to directly build a flat-field from his data, depending on the
brightness of the background, and the relative extension of the
target source(s) with respect to the raster (or beam-switch)
steps. In all staring mode observations, as well as in a few cases of
raster and beam-switch observations, particularly of very extended
sources or crowded fields, the use of a flat-field library is nevertheless
unavoidable.
There have been two kinds of flat-field observations:
is a matrix with 1's everywhere.
Flat-fielding is a crucial step in the data processing, in particular
in the infrared, where the brightness of the background is very often
comparable to (or higher than) the brightness of the target
source. Therefore, the generation of flat-fields
is a very important step in
the overall calibration strategy of ISOCAM.
It must be noted,
however, that many observations in raster and beam-switch mode allow
the observer to directly build a flat-field from his data, depending on the
brightness of the background, and the relative extension of the
target source(s) with respect to the raster (or beam-switch)
steps. In all staring mode observations, as well as in a few cases of
raster and beam-switch observations, particularly of very extended
sources or crowded fields, the use of a flat-field library is nevertheless
unavoidable.
There have been two kinds of flat-field observations:
- observations of the Zodiacal Background, which we will refer to
as `ZB flat-fields' in the following;
- observations of the Internal Calibration Device,
which we will refer to as `ICD flat-fields'.
The targets for ZB observations were chosen as close as possible to
the sun-viewing constraint of ISO in order to maximise their flux.
The choice of the raster strategy for observation allows the
minimisation of possible non-uniformities in the zodiacal background,
since a given pixel of the detector sees different sky pixels during
the raster, and a flat-field image can be constructed from the median of all
raster-position images. A total of  100 ZB
raster observations were executed in the available ISOCAM calibration
time.
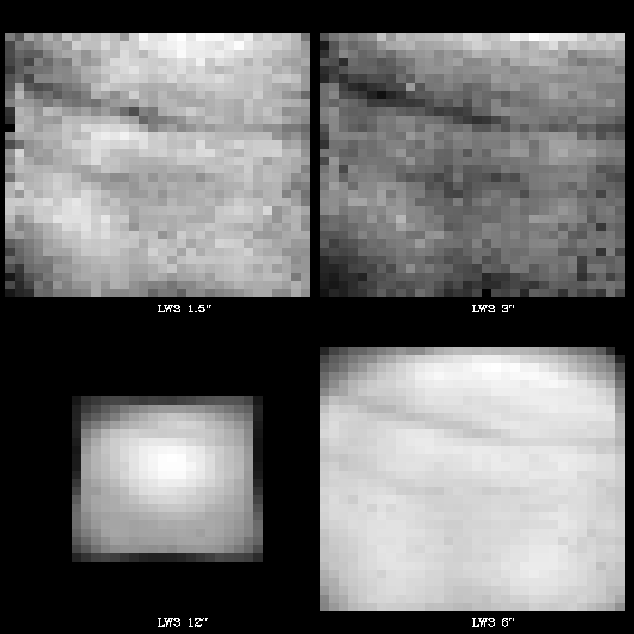
In Figure 4.13 we show four examples of ZB flat-fields
in the LW3 (15
100 ZB
raster observations were executed in the available ISOCAM calibration
time.
In Figure 4.13 we show four examples of ZB flat-fields
in the LW3 (15 m) filter, with 1.5
m) filter, with 1.5
 ,
3
,
3
 , 6
, 6
 and
12
and
12
 pfov, respectively.
It can be seen that only the central part of the array is illuminated
when the 12
pfov, respectively.
It can be seen that only the central part of the array is illuminated
when the 12
 pfov is chosen. The pixel dependent
stabilisation times generate a pattern across the images which
increases the spatial noise. Low frequency noise levels can be
observed for several minutes after a flux step. The main problems are
for pixels located at the edges of the array, and on a line in the
lower left hand quadrant of the array which has a slightly lower
responsivity than the average (and it is usually nicknamed: `the
hair'). The flat-fields have been normalised so that the mean of the
central 11
pfov is chosen. The pixel dependent
stabilisation times generate a pattern across the images which
increases the spatial noise. Low frequency noise levels can be
observed for several minutes after a flux step. The main problems are
for pixels located at the edges of the array, and on a line in the
lower left hand quadrant of the array which has a slightly lower
responsivity than the average (and it is usually nicknamed: `the
hair'). The flat-fields have been normalised so that the mean of the
central 11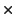 11 pixel sub-array is one. This definition has the
advantage that vignetted outer pixels do not alter the normalisation
factor, causing the flat-field to directly contribute to the
photometric calibration factor for the system. (Ideally, the flat-
field should only adjust spatial fluctuations, it should not affect
the average signal level for the array.)
11 pixel sub-array is one. This definition has the
advantage that vignetted outer pixels do not alter the normalisation
factor, causing the flat-field to directly contribute to the
photometric calibration factor for the system. (Ideally, the flat-
field should only adjust spatial fluctuations, it should not affect
the average signal level for the array.)
Figure 4.13:
Four examples of zodiacal background flat-fields in the LW3 filter.
From top-left in clockwise order: 1.5
 ,
3
,
3
 , 6
, 6
 ,
12
,
12
 pfov,
respectively. Note that in the case of the 12
pfov,
respectively. Note that in the case of the 12
 pfov, only part of the array is illuminated.
pfov, only part of the array is illuminated.
|
|
Even with a careful choice of the brightest zodiacal positions, the
zodiacal background does not provide enough illumination for a
proper calibration of all ISOCAM configurations. This is the case
for the shorter-wavelength, small-pfov, configurations of CAM LW, and
for all of the SW configurations. For LW a solution was adopted
to replace the flat-field of configurations with low zodiacal
background with the ZB flat-field of
a corresponding configuration nearest in wavelength. The only
solution for CAM SW was to use the ICD as the calibration source. This
choice has the drawback that the ICD does not provide a uniform
illumination of the field of view of ISOCAM, and considerable
vignetting is seen at the edges of the detector. (See
Section 2.7).
It is important to stress again that most raster and beam-switch
ISOCAM scientific observations can provide independent flat-field
estimations. The idea behind these observing modes was indeed to
beat the pixel-to-pixel response variation by observing the same sky
region with different pixels. Observers using these observing modes
do not need to be particularly concerned about the quality of the
calibration flat-fields. The calibration flat-fields are however
necessary for staring observations (or when the source is very extended
with respect to the raster dimensions, or the observed field is very
crowded).
Imperfect flat-fielding induces two kind of error:
- photometric
error arising from the uncertainty in the responsivities of the
pixels that see each source; and
- the photometric error arising from the background subtraction.
As the source will be usually located at
the centre of the array, the former error will always be relatively
low, of the order of a few percent, even when no sky flat-field is
available,
since one can safely use the ICD flat-field near the array centre. On the
other hand, identifying faint sources over a high-brightness
background requires a very accurate flat-field.
As an example, the currently achieved median accuracy of 0.5% in
the LW10 6
 flat-field
means that with an average background
flux of 50 ADU/G/s in this configuration, the 1-
flat-field
means that with an average background
flux of 50 ADU/G/s in this configuration, the 1-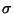 limit for the
detection of a faint source per pixel is
limit for the
detection of a faint source per pixel is 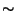 60
60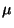 Jy (using
the ADU/G/s to Jy conversion factors from Blommaert 1998,
[10]).
On the other
hand, the poor accuracy of only 6% that we have reached for the
LW2 1.5
Jy (using
the ADU/G/s to Jy conversion factors from Blommaert 1998,
[10]).
On the other
hand, the poor accuracy of only 6% that we have reached for the
LW2 1.5
 flat-field gives a 1-
flat-field gives a 1-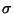 limit per pixel of
limit per pixel of
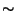 20
20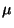 Jy for a typical background flux of 0.8 ADU/G/s in this
configuration (note however that in this low background configuration
one needs a long measurement to reduce the readout noise). In other
words, in the configurations where the
background flux is high we need a higher flat-field accuracy, but if the
flux is high, a higher ZB flat-field accuracy is easier to achieve. The
typical flat-field accuracy is about 1-3%.
Jy for a typical background flux of 0.8 ADU/G/s in this
configuration (note however that in this low background configuration
one needs a long measurement to reduce the readout noise). In other
words, in the configurations where the
background flux is high we need a higher flat-field accuracy, but if the
flux is high, a higher ZB flat-field accuracy is easier to achieve. The
typical flat-field accuracy is about 1-3%.




Next: 4.6 Point Spread Function
Up: 4. Calibration and Performance
Previous: 4.4 Transients
ISO Handbook Volume II (CAM), Version 2.0, SAI/1999-057/Dc